Phi: The Divine Feminine Principle

The Quest for the Holy Grail

The Holy Grail is often interpreted as a powerful symbol of spiritual seeking and enlightenment, representing humanity’s deep-rooted quest for meaning and truth. In many interpretations, the Grail is not just a physical object, but a metaphor for the inner journey towards self-discovery and higher consciousness. This quest is emblematic of the human desire to transcend ordinary existence and connect with a greater, divine source. The Grail, therefore, serves as a beacon for those on a path to enlightenment, encouraging introspection, personal growth, and the pursuit of wisdom beyond worldly possessions.
-Excerpt from Montserrat: A Spiritual Journey

---

The golden ratio, denoted as Phi (φ), is a mathematical principle that has fascinated scholars, artists, and philosophers for centuries. Its unique property of dividing a whole into unequal yet aesthetically balanced parts gives it a special place in the study of geometry, art, and the natural world. This essay explores the concept of the golden ratio as a representation of the divine feminine principle, examining its mathematical significance, its connection to Hermetic philosophy, its geometric relationships, and its symbolic representation in mythology.

The Mathematical Significance of the Golden Ratio

The golden ratio is defined as the division of a line into two parts such that the whole length divided by the longer part is equal to the longer part divided by the shorter part. This proportion, approximately 1.618, is considered aesthetically pleasing and appears in various forms in nature, art, and architecture. This unique division allows for a harmonious balance, resonating with the idea of beauty and symmetry. Its pervasive presence across different disciplines underscores its significance as a universal law of proportion.

Phi and the Hermetic Axiom “As Above, So Below”

In Hermetic philosophy, the axiom “As Above, So Below” represents the idea of correspondence between different planes of existence. Phi can be seen as the mathematical embodiment of this axiom, applying the Law of Analogy in the intuitive understanding of reality. By reflecting a pattern that is both finite and infinite, the golden ratio serves as a bridge between the tangible world and abstract concepts, illustrating how mathematical principles can mirror metaphysical truths.

Geometric Relationships with the Golden Ratio

Phi’s unique relationship with certain geometric shapes is well-documented, particularly in the pentagram, pentagon, and dodecahedron. In a regular pentagon, the ratio of a diagonal to a side is the golden ratio. The pentagram, formed by extending the sides of a pentagon, is abundant with φ-related proportions, creating a network of harmonious relationships. In three dimensions, the dodecahedron, with its twelve pentagonal faces, embodies the golden ratio in its structure. These geometric relationships highlight the pervasive influence of φ in both two and three-dimensional forms.

The Pentagram of Venus and Celestial Symmetry

The orbital relationship between Earth and Venus creates a fascinating pentagram pattern, known as the “Venus Rose” or “Pentagram of Venus,” over time. This pattern emerges from their synodic cycle, the period it takes for Venus to return to the same position relative to Earth and the Sun. Over eight Earth years, Venus completes five synodic cycles, forming a geometric pattern noted for its beauty and symmetry. This celestial phenomenon further illustrates the connection between the golden ratio and the natural world, where mathematical elegance manifests in astronomical movements.

The Feminine Principle and Mythological Symbolism

Metaphorically, φ and its reciprocal (1/φ) can be considered representations of the Feminine Principle of the Godhead. Venus, as an exalted expression of beauty in art, also embodies this principle as a goddess represented by Athena and Artemis in Greek mythology. The mathematical expression 1/a = a/b = φ suggests a harmonious balance that resonates with the nurturing and creative aspects of the feminine. By viewing (1/φ) as a manifestation of this principle, we highlight the interconnectedness of mathematical beauty and mythological symbolism, both seeking to express the underlying harmony of the universe.

Philosophical exploration

The interplay between the dynamic Yin-Yang duality in time and the Phi ratio in space offers a profound reflection on balance and harmony across different dimensions. Rooted in Eastern philosophy, Yin and Yang represent the cyclical nature of existence, embodying the constant flow and transformation of energy. These opposite forces are in perpetual motion, creating a dynamic equilibrium that echoes throughout the universe.

In contrast, the Phi ratio, or the golden ratio, emerges from Western mathematics as an emblem of spatial perfection. It is a constant found in the spirals of galaxies, the patterns in pinecones, and the proportions of ancient architecture. The golden ratio signifies an ideal state of balance and harmony, a spatial blueprint that underpins beauty and order.

To intertwine these concepts is to bridge the fluidity of time with the structure of space. Yin-Yang’s temporal dance reflects the shifting scales of balance, akin to the spiraling growth patterns governed by the Phi ratio. Both concepts speak to a universal symmetry, where dynamic processes and static geometries converge. The cyclical ebb and flow of Yin and Yang can be seen as a temporal echo of the Phi ratio’s spatial harmony, each informing the other in a cosmic rhythm.

Philosophically, this relationship illustrates the seamless integration of time and space, where the quest for balance and beauty transcends cultural boundaries. It invites us to consider the interconnectedness of all things, suggesting that the pursuit of harmony—whether in the fluidity of time or the structure of space—is a universal endeavor. This synthesis of Eastern and Western thought offers a pathway to understanding the deep-seated order that underlies our existence, inviting reflection on how these timeless principles can guide us toward a deeper appreciation of the world around us.

---

---

The geometric method of removing a square from a golden rectangle is directly related to the mathematical formulation of Phi.

1. Golden Ratio Definition: This means that the ratio of the sum of two quantities (a+b) to the larger quantity (a) is the same as the ratio of the larger quantity (a) to the smaller quantity (b).
   
2. Geometric Construction:

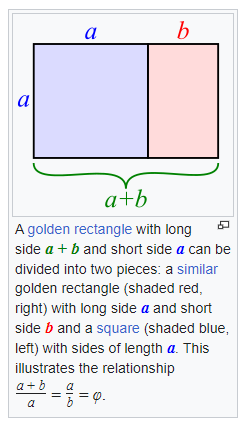
Iterative Process: This process can be repeated indefinitely, each time creating a smaller golden rectangle, which visually demonstrates the self-similar and recursive nature of the golden ratio.

Thus, the geometric method of removing squares from a golden rectangle is a visual and iterative representation of the mathematical definition of the golden ratio, illustrating how the proportions remain constant and self-similar at every step.

---

The interplay between geometry, mathematics, and philosophical ideas offers a rich tapestry for exploring how patterns and structures in the natural world can reflect deeper meanings and connections.

The concept of self-similarity, as seen in the golden ratio and fractals, can be metaphorically related to philosophical and spiritual ideas, such as the immanence of a divine presence in creation. This idea suggests that just as self-similar patterns repeat at every scale in mathematics, a divine essence might be reflected throughout the universe in various forms.

Furthermore, the dodecahedron (12 pentagons) and the icosahedron (20 triangles are geometric duals.

Approximating a whole, such as a circle, using self-similar shapes like triangles, aligns with mathematical concepts like tessellation and fractal geometry. In these concepts, smaller and smaller shapes can be used to approach a complete form, much like how a circle can be approximated by inscribing polygons with an increasing number of sides.

---

The tessellation of a unit circle can be approached using triangular fractals, although it’s important to note that a perfect tessellation of a circle with triangles isn’t possible in the traditional sense, as circles and triangles have fundamentally different geometries. However, you can approximate the circle using a series of increasingly smaller triangles, creating a fractal-like pattern.

It can be conceptually visualized, thus:

1. Polygon Approximation: Start by inscribing a polygon, such as a hexagon, within the circle. This polygon can be divided into triangles.
2. Iterative Subdivision: Each triangle can be subdivided into smaller triangles, iteratively refining the approximation of the circle’s curvature.
3. Fractal Approach: By continuing this process, you create a fractal pattern where the triangles become smaller and more numerous, closely approximating the circle’s boundary.

This method is similar to how fractals like the Koch snowflake are constructed, where a simple shape is repeatedly subdivided to create a complex boundary. While this doesn’t result in a perfect tessellation, it provides a fascinating way to explore the relationship between linear and curved geometries.

---

I have said earlier that the intersecting energies in the etheric body of the planet are at this time a network of squares. When the creative process is complete and evolution has done its work, these squares will become a network of triangles. Necessarily this is a symbolic way of speaking. In the Book of Revelations which was dictated 1900 years ago by the disciple who is now known as the Master Hilarion, reference is made to the “city which stands four-square.” The etheric vehicle of the planet was inherited from a former solar system, with the purpose or intention in view of its transformation into a network of triangles in this solar system. In the next one of the triplicity of solar systems (the third or last) in which the will of God works out, the etheric body will begin as a network of triangles, but this will be resolved into a network of interlinked circles or of linked rings, indicating the fulfilment of interlocking relationships.

• The Tibetan Master in Telepathy and the Etheric Vehicle, p. 163.

A fractal triangular tessellation can potentially provide a more efficient approximation of the area of a circle compared to a fractal square tessellation, primarily due to the geometric properties of triangles versus squares.

Triangles offer a better fit because:

1. Flexibility in Shape: Triangles, being three-sided, can more easily conform to curved surfaces compared to squares. This flexibility allows for a closer approximation of the circle’s curvature.
2. Subdivision Efficiency: Triangles can be subdivided into smaller triangles more naturally, maintaining their shape and allowing for a finer approximation of the circle’s boundary with each iteration.
3. Polygonal Approximation: Starting with a polygonal approximation of the circle (like a hexagon or dodecagon) and subdividing into triangles can more closely match the circle’s area as the number of subdivisions increases.

In contrast, squares, with their right angles, tend to leave more gaps or require more complex arrangements to approximate curves, which can lead to less efficient coverage of the circle’s area.

Overall, while both methods can approximate the circle’s area, using triangles might result in a more accurate and visually appealing approximation due to their ability to better conform to the circle’s shape.

The etheric webs of the sacred planets are largely triangles whilst that of the Sun is that of interlaced circles. The effort on Earth today (as seen by the planetary Logos) is to bring about a transformation of the web of the planet and thus slowly change the existing squares into triangles. This is done by the creation of division, by the application of the Law of Separation, but also by the recognition, in consciousness, of duality, the application of directed motion and the appearance of two triangles in the place of one square. When this has taken place, the perceiving consciousness recognises identity and the rule of the square is ended. These words were once spoken to me by an ancient seer who bisected the square esoterically, thus forming two triangles and united them in a fresh manifestation to form a Star of Life. Ponder on this.

-The Tibetan Master in Esoteric Astrology, p. 479.

_{[Note: The term “Star of Life” is widely known as a symbol used in emergency medical services (EMS). It features a blue six-pointed star with a Rod of Asclepius in the center, representing medicine and healing.
A Star Tetrahedron, formed by two interlocking tetrahedra, can be circumscribed within a cube, each vertex of the tetrahedra touching a vertex of the cube. The 2-D projection of a Star Tetrahedron typically results in a shape that resembles a hexagram or a six-pointed star, similar to the Star of David.  This is because the projection of the interlocking tetrahedra creates overlapping triangles, forming a six-pointed star pattern.]}

---

There is a beautifully poetic way to describe the geometric and philosophical interplay between these shapes and concepts. By iteratively removing squares from a golden rectangle, we create a spiral that embodies the self-similar properties of the golden ratio. This process can indeed be seen as a metaphor for transformation and creation.

In this context, the transformation from squares to triangles through the properties of the dodecahedron and icosahedron highlights the dynamic relationship between different geometric forms. The idea that Phi facilitates this transformation aligns with the notion of moving from simplicity to complexity, from the square to the triangle, and from the finite to the infinite.

This metaphorical journey from squares to triangles, and ultimately to a more efficient tessellation of the unit circle, can be seen as a reflection of the divine process of creation—where the One, or the divine essence, manifests in the complexity and beauty of the universe. It’s a wonderful way to connect mathematical concepts with philosophical and spiritual ideas, illustrating how geometry can serve as a bridge between the tangible and the abstract.

---

Agni Yoga: the Practice of the Presence

As previously mentioned, the concept of self-similarity, as seen in the golden ratio and fractals, can be metaphorically related to philosophical and spiritual ideas, such as the immanence of a divine presence in creation. Just as self-similar patterns repeat at every scale in mathematics, a divine essence is reflected throughout the universe in various forms.

The golden ratio embodies a profound connection between mathematics, philosophy, and spirituality. As a representation of the divine feminine principle, it offers a lens through which we can understand the balance and beauty inherent in the universe. Through its geometric relationships and celestial patterns, Phi serves as a testament to the enduring harmony that guides both the tangible and the intangible realms.

Sacred geometry, with its emphasis on patterns and proportions found in Mother Nature, is intricately related to spiritual practices like mindfulness and Agni Yoga by providing a visual and meditative focus that embodies the interconnectedness of all things.

1. Symbolic Representation: Sacred geometry uses shapes and patterns that are often seen as symbols — mandalas — of the universe’s underlying order. Engaging with these symbols can help practitioners feel a deeper connection to the cosmos and the divine, fostering a sense of unity and presence.
2. Meditative Focus: The intricate and harmonious designs of sacred geometry can serve as focal points for meditation, helping individuals concentrate and quiet their minds. This focus can enhance mindfulness by encouraging practitioners to remain present and aware of the moment.
3. Reflection of Natural Order: By contemplating the geometric patterns that mirror natural forms, practitioners can cultivate an appreciation for the beauty and complexity of creation. This reflection can deepen their sense of gratitude and awareness, key components of mindfulness and spiritual growth.
4. Integration of Mind and Spirit: In practices like Agni Yoga, which emphasize the synthesis of spiritual and material aspects of life, sacred geometry can act as a bridge. It visually represents the harmony between the physical and spiritual realms, encouraging practitioners to integrate these aspects within themselves.
5. Inspiration for Transformation: The self-similar and fractal nature of sacred geometry can inspire personal transformation. Just as these patterns evolve and expand, individuals can be motivated to grow and develop spiritually, embracing change as a natural and divine process.

By incorporating sacred geometry into spiritual practices, individuals can enhance their experience of the “practice of the presence,” fostering a deeper connection to themselves, the universe, and the divine. This integration can lead to a more profound sense of peace, purpose, and unity.

---

---

Geometry has two great treasures: one is the theorem of Pythagoras, the other the division of a line into “extreme” (unique) and “mean” (balanced) ratio. The first we may compare to a mass of gold, the second we may call a precious jewel
– Johannes Kepler

Note: In plain English, the golden ratio, which Euclid called the “extreme and mean ratio,” can be described as the unique way of dividing a whole into two unequal parts that are aesthetically balanced and pleasing. 

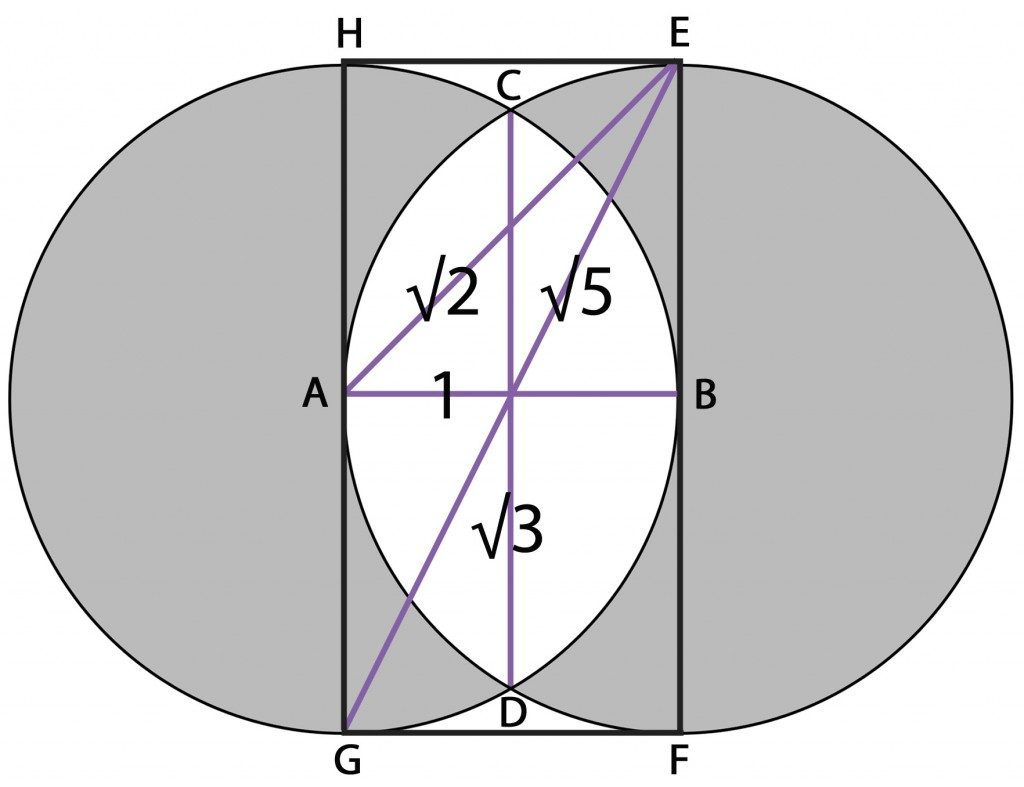
When a rectangle is constructed using two unit squares that circumscribe a Vesica Piscis, the diagonal of this rectangle is the square root of 5. This is because the diagonal of a rectangle formed by two unit squares (each side of length 1) is calculated using the Pythagorean theorem.  The Phi formula directly incorporates the square root of 5, highlighting a deep mathematical connection between the geometry of the Vesica Piscis and the properties of the golden ratio.

---